The content is written for pdf viewer. please refer to the attachment.
Introduction
Nobody would like to be victimized by criminals. Naturally, we are concerned about our security issues to be alive by our instinct as human beings. In this respect, Mustard and Lott’s (1997) study shows that allowing citizens to carry concealed handguns deters violent crimes effectively. However, as the law and the methodology have changed since 1997, this study has become controversial. This is attributed to the fact that the study used county-level data while the law is applied at the state level in reality. This can be a substantial measurement error that falls short of vindicating the research point. Therefore, this paper is designed to redeem the weakness of the Mustard and Lott study.
Background and Economic Theory
“Shall issue” law means that individuals must have a license to carry a concealed handgun, and the granting of such licenses is subject only to meeting specific criteria[1]. Namely, the law made concealed handguns legal. Mustard and Lott investigated the deterrence effect of “shall issue” right-to-carry concealed handgun laws. The methodology used new data, annual cross-sectional time-series county-level crime data of the US from 1977 to 1992.
Table SEQ Table \* ARABIC 1: The Handgun Laws state by state between 1977 and 1992
| Already had the laws | Newly adopted laws | Special case |
| Alabama Connecticut Indiana, New Hampshire North Dakota South Dakota Vermont Washington |
Florida (1987) Georgia (1989) Idaho(1990) Maine (1985) Mississippi (1990) Montana (1991) Oregon(1990) Pennsylvania (1989) Virginia (1988) West Virginia (1989) |
Pennsylvania |
The states in ‘Already had the laws’ indicate the states whose firearm laws existed before 1977, whereas ‘Newly adopted laws’ mean states whose laws are newly applied given years for each. Pennsylvania is an exceptional case in that Philadelphia was exempted from the state law. The rest of the states not listed in this table imply that the laws are not applicable.
[1] Shall Issue States 2022, World Population Review, 2022, https://worldpopulationreview.com/state-rankings/shall-issue-states.
The economic theory of the deterrence effect of the law is simply constructed from the extended concept of opportunity cost. To be more specific, the victim directly encounters the criminal in a circumstance where the victim can do self-defense as a counterattack against firearmed criminals. In this case, the probability of receiving opportunity cost as death to criminals is increased by the victims and police. This concept can be linked to other economic theories. One of the examples is Game Theory. Given two players, the victim(V) and the criminal(C), (V) has two actions between firearms, and none, and (C) has two actions between committing a crime and doing nothing. Even though both players cannot observe the actions of each in advance, (C) knows the “shall issue” law led to an increase in the probability of action firearms by (V) with the lowest payoffs(death) to (C) as an outcome. Naturally, the law deters the action of committing a crime by (C) to avoid the lowest payoffs, death. Furthermore, this concept can also link to another economic concept based on the price of obtaining guns procedure variation by the law and other theories.
Mustard and Lott argued that previous studies ignored the heterogeneity within
States, and thus, they have biased results. That is, there are unaffected counties by the law that should be excluded to precisely analyze the deterrence effect of the law. This assertion implies that Mustard and Lott suggest a more accurate analysis of the deterrence effect of the law on a county basis with new time-series data, unlike previous papers.
Data
In this paper, the data set originated from The FBI's Crime Data Explorer (CDE). The data is the number of recorded crimes from 1979 to 2020 by all states and crime types. In order to figure out the effect of the “shall issue” law, this section selects the state which adopted the law in 1989 (Georgia, Pennsylvania, and West Virginia) and 1990 (Idaho, Mississippi, and Oregon) as samples for better understandings to readers with a convenient purpose. Since the original measurement for ‘rape’ was abolished in 2017 and a new measurement for ‘rape’ has been adopted, the data in this paper covers from 1979 to 2016 only for consistency. Highlighted yellow cells indicate the year when the “shall issue” law was adopted. The sample table consists of the sum of three states’ crimes. This section picks homicides as a representative crime type to compare the trend by the states in the Figures. Note that this analysis based on simplified sample data is extended to the entire data in the section from the bacon decomposition.
Table 2: Sum of crime types from Georgia, Pennsylvania, and West Virginia
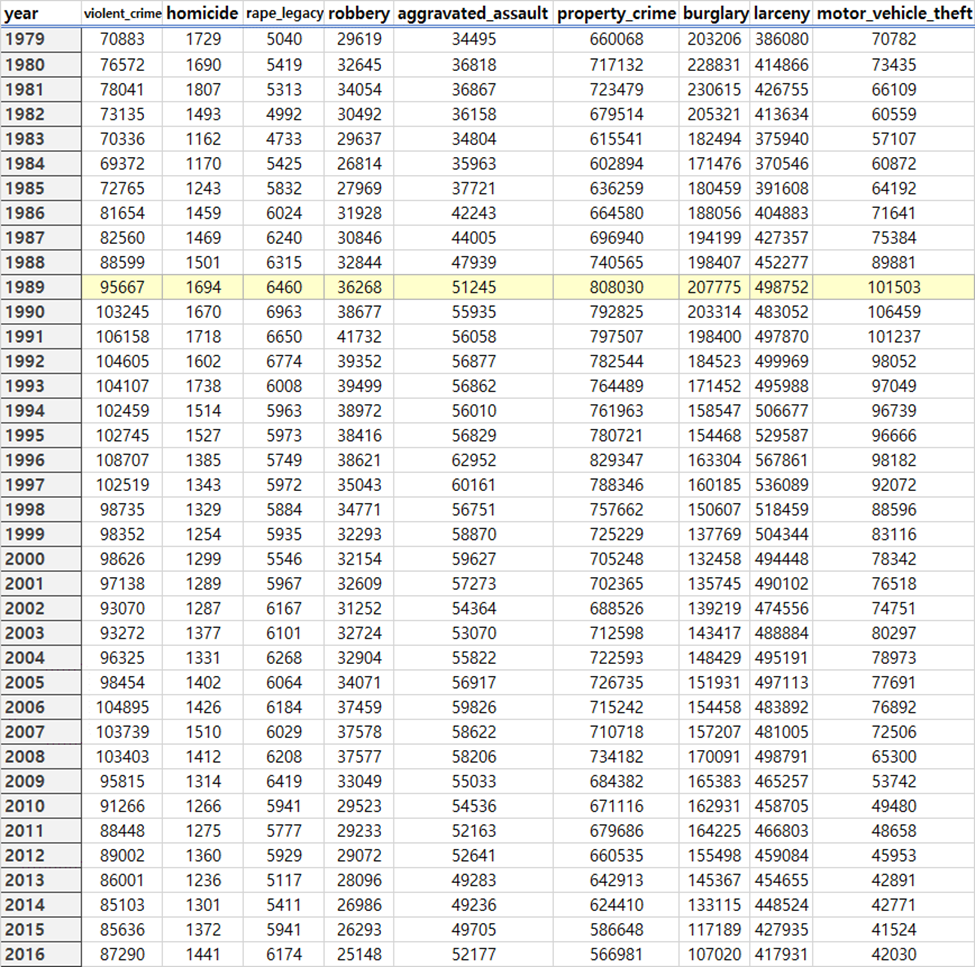
The table shows that the adopted law did not significantly decrease the number of all types of crimes instantly in 1990. Property crime, burglary, and larceny show a slightly decreasing in 1990. Then, consider the plot graph of homicide trends in the separate states.
Figure 1: Homicide Trend (law enacted in 1989)

Although the instant effect of the law is not necessarily guaranteed in 1991, the Figure shows the existence of a gradual decrease from 1990 to 2000.
Table 3: Sum of crime types from Idaho, Mississippi, and Oregon

In this table, the increasing crimes in 1991 appear after the adopted law in 1990. Like the table above, the instant effect was not necessarily guaranteed.
Figure 2: Homicide Trend (law enacted in 1990)

The figure shows that the decreasing trend appears after 1994 while increasing from 1990 to 1994.
Empirical model and Estimation
This section applies data analysis with regressions in four ways: Two-way fixed effects (TWFE), Bacon decomposition, Callaway and Sant’anna, and Event study. Note that ‘crime_rate’ is the outcome of regression and is equal to the sum of all crime types divided by population.
1. Two-way fixed effects (TWFE)
TWFE is applied separately to the group of the law enacted in 1989 and in 1990 as the prime examples. In order to clarify the effect of the law, the regression periods are divided by the year the law was enacted. For example, the first estimate (~1989) from Table 4 indicates the period from 1979 to 1989, while the second estimate (1989~) indicates the period from 1989 to 2016. Both estimates include the year of the enacted law. The detailed information, such as standard errors, corresponds to the new estimates only. Note that ‘motor_vehicle_theft’ and ‘aggravated_assault’ for old estimates are dropped because of multi-collinearity.
Table 4: TWFE (1989) for Georgia, Pennsylvania, and West Virginia
| Term | Estimate (~1989) | Estimate (1989~) |
std.error | Statistic | p.value |
| violent_crime | 0.0002231 | 0.0012206 | 0.0007228 | 1.6886581 | 0.0983611 |
| Homicide | -0.0029738 | -0.0095062 | 0.0016221 | -5.8603355 | 0.0000005 |
| rape_legacy | -0.0001212 | -0.0025675 | 0.0008128 | -3.1586808 | 0.0028633 |
| Robbery | -0.0002060 | -0.0004848 | 0.0007391 | -0.6560197 | 0.5152275 |
| aggravated_assault | N/A | -0.0012449 | 0.0007273 | -1.7117891 | 0.0939767 |
| property_crime | 0.0000296 | -0.0000250 | 0.0000262 | -0.9539615 | 0.3453149 |
| Burglary | 0.0000766 | 0.0000666 | 0.0000377 | 1.7638924 | 0.0846926 |
| Larceny | 0.0000541 | 0.0000793 | 0.0000310 | 2.5594881 | 0.0139957 |
In the table, all of the observed coefficients of variables are decreased except for larceny after enacting the law in 1989. This implies that ‘crime_rate’ is comparatively low than in the previous period.
Table 5: TWFE (1990) for Idaho, Mississippi, and Oregon
| Term | Estimate (~1990) |
Estimate (1990~) |
std.error | Statistic | p.value |
| violent_crime | -0.0000835 | 0.0011512 | 0.0008028 | 1.4339656 | 0.1583452 |
| Homicide | 0.0023399 | 0.0062640 | 0.0030716 | 2.0393563 | 0.0471830 |
| rape_legacy | 0.0017381 | 0.0002652 | 0.0009212 | 0.2878448 | 0.7747585 |
| Robbery | 0.0001856 | -0.0013858 | 0.0007941 | -1.7450454 | 0.0876548 |
| aggravated_assault | N/A | -0.0009862 | 0.0007902 | -1.2481267 | 0.2183005 |
| property_crime | 0.0000047 | 0.0000133 | 0.0000239 | 0.5571714 | 0.5801115 |
| Burglary | -0.0000125 | 0.0000134 | 0.0000353 | 0.3801357 | 0.7055943 |
| Larceny | 0.0000209 | 0.0000342 | 0.0000347 | 0.9873099 | 0.3286557 |
In the table, the positive effect of the law is limited to the crime types of ‘rape_legacy’ and ‘robbery’ only. This result questions the effectiveness of the “shall issue” law.
2. Bacon decomposition
Unlike the TWFE section above, this section uses the entire data containing all states with the same concept of ‘crime_rate.’ Moreover, the data uses numeric variables of ‘state_name’ and binary treatment variable. The yardstick of the treatment variable is the year when the law was enacted, so that '1' means treated status. For example, enacted year of Georgia, Pennsylvania, and West Virginia is 1989. Then the treatment variable for 1979 to 1988 is ‘0’ whereas the year from 1989 to 2016 is ‘1.’ This treatment variable contains omitted states by Mustard and Lott referenced to the historical facts[2]. Furthermore, it includes the updated fact, such as Illinois adopted the “shall issue” law in 2013. As seen the Table 6 below, the coefficient of treatment variable for TWFE is calculated based on this entire data.
Table 6: Bacon Decomposition with TWFE = 0.616128
| Type | Weight | Estimate | |
| Earlier vs Later | Treated | 0.18044 | 0.16362 |
| Later vs Always | Treated | 0.63998 | 0.88175 |
| Later vs Earlier | Treated | 0.17958 | 0.12419 |
[2] Higgins, P., Owens, S., Woodward, M., & Wayne. (2022, January 29). The ultimate concealed carry guide (2019). GunsToCarry. Retrieved May 26, 2022, from https://www.gunstocarry.com/
Figure 3: Bacon Decomposition with TWFE = 0.616128

This bacon decomposition shows how much TWFE can be biased by representing the variance weight of the estimate. In this decomposition, the ‘Later vs always treated’ group has the most significant weight. The biggest problem with TWFE is that it uses the treated group as a control group (untreated group), which is the leading cause of bias. Even though this result does not include the case of the sign flipped, considering the fact that TWFE does not have “no sign flip property,” this method can implicitly arise severe misleading results. This problem induces to use of other better estimating methods such as Callaway and Sant’anna. (CS)
3. Callaway and Sant’anna (CS)
Using the CS method (‘did’ package in R) estimates the effect on ‘crime_rate.’ The panel time variable is ‘year,’ and the panel id variable is ‘sid’ which is a converted numeric variable from the columns ‘state_name.’ The first treatment period variable is the ‘enacted_year’ for each ‘sid.’ The estimation method is doubly robust and it does not set any covariates. Then the equation for this method would be:


The result from ‘did’ library package in R is below:
| Call: att_gt(yname = “crime_rate", tname = "year", idname = "sid", gname = "enacted_year", xformla = NULL, data = crime, panel = TRUE, control_group = "notyettreated", bstrap = TRUE, biters = 1000, est_method = "dr", print_details = FALSE) Reference: Callaway, Brantly and Pedro H.C. Sant'Anna. "Difference-in-Differences with Multiple Time Periods." Journal of Econometrics, Vol. 225, No. 2, pp. 200-230, 2021. <https://doi.org/10.1016/j.jeconom.2020.12.001>, <https://arxiv.org/abs/1803.09015> Group-Time Average Treatment Effects: |
|
| Group Time ATT(g,t) Std. Error [95% Simult. Conf. Band] 1985 1980 -0.5093 0.0892 -1.2381 0.2194 1985 1981 -0.1817 0.0988 -0.9893 0.6258 1985 1982 -0.3257 0.1135 -1.2533 0.6019 1985 1983 0.3515 0.1004 -0.4695 1.1726 1985 1984 -0.0924 0.0544 -0.5368 0.3519 1985 1985 -0.1011 0.1010 -0.9266 0.7244 1985 1986 -1.0315 0.1598 -2.3374 0.2744 1985 1987 -0.9144 0.1792 -2.3796 0.5508 1985 1988 -0.7936 0.2386 -2.7441 1.1569 1985 1989 -0.8279 0.2851 -3.1583 1.5025 1985 1990 -0.9699 0.3023 -3.4406 1.5008 1985 1991 -1.1607 0.2845 -3.4861 1.1646 1985 1992 -1.2145 0.2893 -3.5796 1.1507 1985 1993 -1.7586 0.3056 -4.2569 0.7396 1985 1994 -1.4979 0.3055 -3.9948 0.9989 1985 1995 -1.3384 0.4300 -4.8537 2.1769 1985 1996 -0.5281 0.4840 -4.4847 3.4286 1985 1997 -1.0537 0.5331 -5.4117 3.3044 1985 1998 -0.8650 0.5471 -5.3374 3.6074 1985 1999 -0.4488 0.5564 -4.9972 4.0996 1985 2000 -0.7252 0.5917 -5.5622 4.1118 1985 2001 -1.0922 0.4108 -4.4501 2.2656 1985 2002 -0.9992 0.4115 -4.3632 2.3648 1985 2003 -1.0212 0.5411 -5.4445 3.4020 1985 2004 -0.7788 0.8000 -7.3184 5.7607 1985 2005 -0.5901 0.6600 -5.9852 4.8050 1985 2006 0.6032 0.5702 -4.0576 5.2639 |
1985 2007 0.5616 0.5873 -4.2392 5.3624 1985 2008 0.8127 0.4899 -3.1916 4.8169 1985 2009 1.0345 0.5441 -3.4127 5.4818 1985 2010 1.3689 0.5239 -2.9137 5.6515 1985 2011 2.6877 NA NA NA 1985 2012 2.8231 NA NA NA 1987 1980 0.8415 0.0850 0.1469 1.5361 * 1987 1981 -0.6885 0.0982 -1.4912 0.1141 1987 1982 -0.7053 0.1089 -1.5957 0.1850 1987 1983 -0.6931 0.0963 -1.4799 0.0937 1987 1984 0.3083 0.0530 -0.1245 0.7411 1987 1985 1.1546 0.0929 0.3948 1.9144 * 1987 1986 0.8264 0.0763 0.2023 1.4505 * 1987 1987 0.4586 0.0974 -0.3380 1.2552 1987 1988 1.3575 0.1525 0.1108 2.6042 * 1987 1989 1.0657 0.2036 -0.5983 2.7297 1987 1990 0.6753 0.2035 -0.9885 2.3392 1987 1991 -0.1294 0.2232 -1.9540 1.6951 1987 1992 -0.0728 0.2296 -1.9499 1.8043 1987 1993 0.1079 0.2556 -1.9815 2.1973 1987 1994 -0.1012 0.3007 -2.5596 2.3571 1987 1995 -1.2552 0.3157 -3.8355 1.3251 1987 1996 -1.1917 0.3702 -4.2178 1.8344 1987 1997 -1.6436 0.4145 -5.0317 1.7444 1987 1998 -2.0445 0.4495 -5.7190 1.6299 1987 1999 -2.6576 0.4517 -6.3504 1.0351 1987 2000 -3.4454 0.5021 -7.5496 0.6588 1987 2001 -4.1196 0.4085 -7.4591 -0.7800 |
| 1987 2002 -4.2509 0.4119 -7.6177 -0.8842 * 1987 2003 -4.6762 0.4486 -8.3435 -1.0089 * 1987 2004 -4.8758 0.5626 -9.4748 -0.2768 * 1987 2005 -5.0607 0.5600 -9.6382 -0.4831 * 1987 2006 -4.3619 0.6509 -9.6829 0.9591 1987 2007 -3.9947 0.6681 -9.4557 1.4664 1987 2008 -3.7394 0.5706 -8.4039 0.9250 1987 2009 -4.1534 0.6248 -9.2609 0.9541 1987 2010 -4.6918 0.6047 -9.6347 0.2510 1987 2011 -3.4688 NA NA NA 1987 2012 -3.8046 NA NA NA 1989 1980 -0.1964 0.0964 -0.9844 0.5915 1989 1981 0.1092 0.1148 -0.8295 1.0478 1989 1982 -0.0545 0.2038 -1.7207 1.6117 1989 1983 -0.0095 0.3673 -3.0117 2.9926 1989 1984 0.0746 0.0860 -0.6280 0.7772 1989 1985 -0.0577 0.4527 -3.7582 3.6427 1989 1986 -0.2153 0.2102 -1.9336 1.5031 1989 1987 0.1008 0.2652 -2.0669 2.2685 1989 1988 0.4764 0.3318 -2.2355 3.1883 1989 1989 0.6179 0.4187 -2.8051 4.0409 1989 1990 0.1800 0.1474 -1.0248 1.3848 1989 1991 -0.0964 0.1934 -1.6775 1.4846 1989 1992 0.1331 0.2252 -1.7080 1.9742 1989 1993 0.0543 0.2991 -2.3904 2.4989 1989 1994 0.0568 0.4143 -3.3299 3.4436 1989 1995 -0.1137 0.3754 -3.1828 2.9553 1989 1996 0.7159 0.3118 -1.8329 3.2646 1989 1997 0.2770 0.5659 -4.3493 4.9033 1989 1998 0.3749 0.7612 -5.8478 6.5975 1989 1999 0.9227 0.9867 -7.1426 8.9880 1989 2000 0.7340 1.1492 -8.6597 10.1277 1989 2001 0.2580 1.2165 -9.6862 10.2022 1989 2002 0.2377 1.2629 -10.0860 10.5615 1989 2003 0.4958 1.1081 -8.5626 9.5541 1989 2004 0.9382 1.2893 -9.6010 11.4773 1989 2005 1.1009 1.3441 -9.8866 12.0884 1989 2006 1.5398 1.5182 -10.8706 13.9502 1989 2007 1.5537 1.5413 -11.0454 14.1529 1989 2008 1.8862 1.3969 -9.5327 13.3052 1989 2009 1.8250 1.5283 -10.6682 14.3183 1989 2010 1.7298 1.4668 -10.2602 13.7197 1989 2011 3.2094 2.1232 -14.1466 20.5655 1989 2012 3.4390 2.7235 -18.8242 25.7021 1990 1980 0.2891 0.1412 -0.8653 1.4436 1990 1981 0.2419 0.3135 -2.3208 2.8047 1990 1982 -0.1505 0.3302 -2.8495 2.5485 |
1990 1983 0.1090 0.1265 -0.9252 1.1432 1990 1984 -0.0166 0.1078 -0.8975 0.8644 1990 1985 0.2545 0.1959 -1.3467 1.8557 1990 1986 -0.0259 0.1728 -1.4381 1.3863 1990 1987 -0.1527 0.1411 -1.3058 1.0005 1990 1988 0.0785 0.1970 -1.5319 1.6888 1990 1989 -0.8628 0.5560 -5.4081 3.6825 1990 1990 -0.3260 0.5255 -4.6213 3.9693 1990 1991 -0.1517 0.6534 -5.4928 5.1894 1990 1992 0.2348 0.6207 -4.8387 5.3082 1990 1993 0.3828 0.7714 -5.9230 6.6887 1990 1994 1.2799 0.8073 -5.3189 7.8787 1990 1995 1.2980 0.3906 -1.8951 4.4911 1990 1996 1.0393 0.6805 -4.5234 6.6021 1990 1997 1.2340 0.6768 -4.2986 6.7666 1990 1998 0.8847 0.8161 -5.7866 7.5559 1990 1999 0.7507 1.1217 -8.4181 9.9196 1990 2000 0.7269 1.0276 -7.6735 9.1273 1990 2001 0.6365 1.0864 -8.2440 9.5170 1990 2002 0.7011 1.1369 -8.5925 9.9947 1990 2003 0.7914 0.9517 -6.9877 8.5705 1990 2004 0.7346 0.9682 -7.1795 8.6487 1990 2005 0.5339 0.9715 -7.4077 8.4756 1990 2006 0.4387 1.3824 -10.8617 11.7391 1990 2007 0.3328 1.4352 -11.3992 12.0648 1990 2008 0.1125 1.2403 -10.0263 10.2513 1990 2009 0.2215 1.3487 -10.8034 11.2463 1990 2010 0.3997 1.3357 -10.5186 11.3181 1990 2011 1.9768 2.3983 -17.6276 21.5813 1990 2012 2.0459 2.0911 -15.0475 19.1393 1991 1980 0.5315 0.0910 -0.2127 1.2758 1991 1981 0.0636 0.0995 -0.7496 0.8769 1991 1982 -0.9502 0.1047 -1.8061 -0.0944 * 1991 1983 1.3638 0.0904 0.6249 2.1028 * 1991 1984 0.2425 0.0538 -0.1971 0.6821 1991 1985 -0.6173 0.1020 -1.4508 0.2163 1991 1986 -0.6726 0.0794 -1.3221 -0.0232 * 1991 1987 0.1554 0.1023 -0.6809 0.9917 1991 1988 -0.6574 0.0868 -1.3673 0.0525 1991 1989 -0.6492 0.1184 -1.6170 0.3187 [ reached 'max' / getOption("max.print") -- omitted 287 rows ] Signif. codes: `*' confidence band does not cover 0 Control Group: Not Yet Treated, Anticipation Periods: 0 Estimation Method: Doubly Robust |
This result allows finding overall ATT. The ATT in this section cannot be a group-level ATT because there are not enough states per treatment year for the bootstrapping to provide accurate 95% confidence intervals. Hence, note that this ATT is simple aggregate ATT with a uniformly weighted average.
Table 7: ATT comparison with covariate or not
| NO covariate | Covariate with population for each state |
| ATT Std. Error [ 95% Conf. Int.] 0.0688 0.3412 -0.5999 0.7375 |
ATT Std. Error [ 95% Conf. Int.] -0.0225 0.2903 -0.5915 0.5464 |
The left result shows that the “shall issue” law could cause a 6.9% increase in the crime rate. The right result is the ATT with covariate ‘population’ of each state. This suggests another research question as to whether the size of the population for each state can be influential on the overall crime rate or not. According to the ATT result, depending on each state’s population, the enacted law can indirectly cause a -2.3% decrease in the crime rate. In light of these results, the right outcome is a comparatively more appropriate model to estimate the effect of the law because of its less standard error.
4. Event study (SA)
The event study is called Sun and Abraham (SA) method, and its estimator is called the interaction weighted estimator. This estimator is compared with any never-treated groups and the last cohort treated. The method limits the data to use the last cohort and never treated units as controls. Namely, all periods when every state is treated are dropped, unlike TWFE, so that the method can overcome the major bias factor of TWFE. Note that the consistently estimated shares weight coefficients.
Figure 4: Comparison between TWFE and SA with crime types

As seen from Figure 4 above, the pre-trend of TWFE is nearby zero and then gradually closed to the negative estimate over time after the treatment. This violates the parallel trend assumption so that TWFE is not a consistent estimate. In contrast, the pre-trend of SA is primarily negative but has an increasing tendency over time despite event time (year = 0, enacted year) passed. This indicates SA estimates satisfy the parallel trend to some extent.
Overall, ATT of SA indicates the “shall issue” policy causes a 44% increase in the crime rate. However, when all crime types are included in the control groups, This declines to a 30.12% increase in the crime rate. Specifically, Violent crime, homicide, and property crime are restrained to less than -0.001 (-0.1%) estimate. Crime types with positive coefficients, such as rape legacy, robbery, aggravated assault, burglary, larceny, and motor vehicle theft, are not that significant because all of them are restricted to less than 0.0012 (0.12%) estimate.
Conclusion
Table 8: How much does the "shall issue" law influence the crime rate in the U.S
| Methodology | TWFE | CA | CA (with covariate) | SA | SA (with crime types) |
| ATT | 0.616128 (62%) |
0.0688 (6.9%) |
-0.0225 (-2.3%) |
0.439 (44%) |
0.298576 (30.12%) |
This table summarizes the conclusion of the procedure of this paper. First, the TWFE method is comparatively not reliable for this paper due to the violation of the parallel trend assumption demonstrated in Figure 4. The problem is that CA and SA are significantly different from each other. However, the SA method is seemingly more reliable than CA in light of the fact that SA is constructed method to overcome the problematic bias that the traditionally preferred method TWFE has. Therefore, the conclusion is that the “shall issue” policy can contribute to an increase in the crime rate in the U.S by 30% ~ 44% despite individual crime types constrained to some extent. This is presumably because the license for firearms led people to abuse the right to take the law into their own hands.
'Project > Personal' 카테고리의 다른 글
| Local sLLM Chatbot (2) | 2024.06.10 |
|---|---|
| KCB 가계부채위험도 평가모델 개발 (0) | 2024.04.22 |
| Avoid Diabetes to Live Alive (0) | 2023.08.18 |
| Quality of Life (1) | 2023.08.18 |
| The Correlation between Lifestyle or Medical Factors and Diabetes (1) | 2023.08.18 |


